Learn about the basic hydraulic formulas, calculations and parameters that apply to hydraulic engineering and how they determine hydraulic system and component performance.
Basic Hydraulic Principles
Hydraulic engineering is based on an area of physics known as fluid dynamics; this important branch of science deals with the movement of fluids. An understanding of the key principles of fluid dynamics is, therefore, essential for anyone who wishes to build or maintain hydraulic systems.
As a science, we can calculate many of a hydraulic system’s properties and behaviours by using known variables. We’ve collated some of the most important hydraulic formulas and calculations that you’re likely to need here.
 »
»
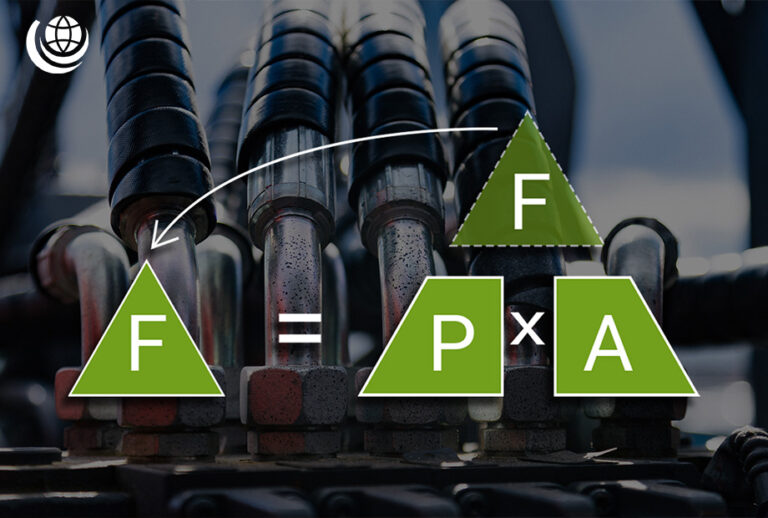
Force, Pressure and Area
F = p x A
p = F / A
A = F / p
When…
F = Force (N)
p = Pressure (Pa)
A = Area (m²)
 »
»
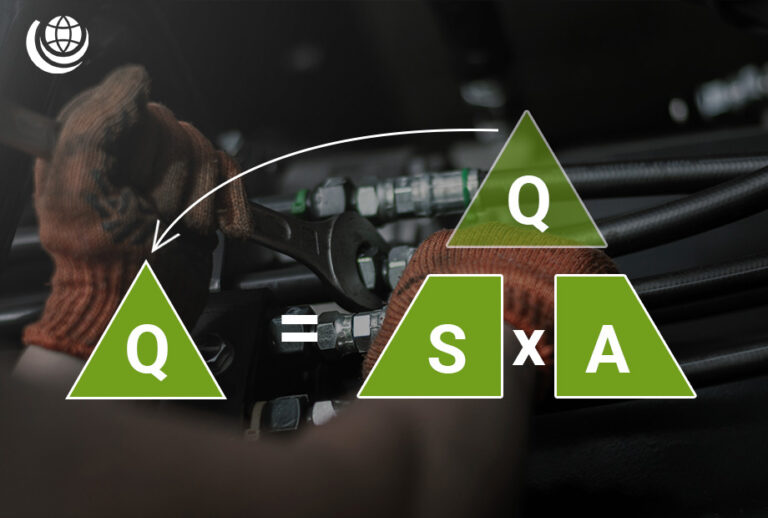
Speed, Flow Rate and Area
Q = S x A
S = Q / A
A = Q / S
When…
S = Speed (cm/s)
Q = Flow Rate (cm³/min)
A = Area (cm²)
Power
P = (Q x p) / 600
when…
P = Power (kW)
p = Pressure (Bar)
Q = Flow Rate (l/min)
600 = Constant
Learn MoreNew to hydraulic terminology? Our Hydraulics Glossary contains over 140 hydraulic terms and definitions used in fluid power engineering…
Hydraulic Formulas for Motors and Pumps
While motor and pumps look similar they actually serve different purposes, pumps use an external power source like an engine or electric motor to provide flow within a hydraulic system. A hydraulic motor on the other hand generates rotational force when fluid flows through it. Here are some formulas you can use to calculate useful figures when working with hydraulic pump and motors.
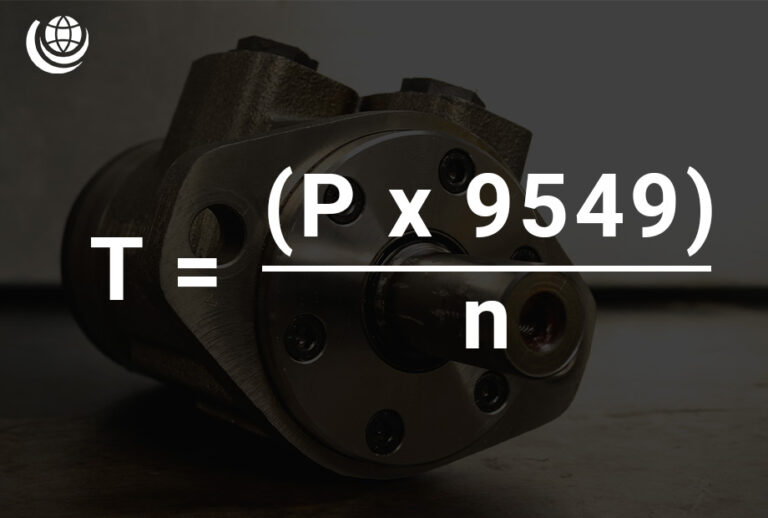 »
»
Torque
T = (P x 9549) / n
When…
T = Torque (Nm)
P = Power (kW)
n = Speed (rpm)
9549 = Constant
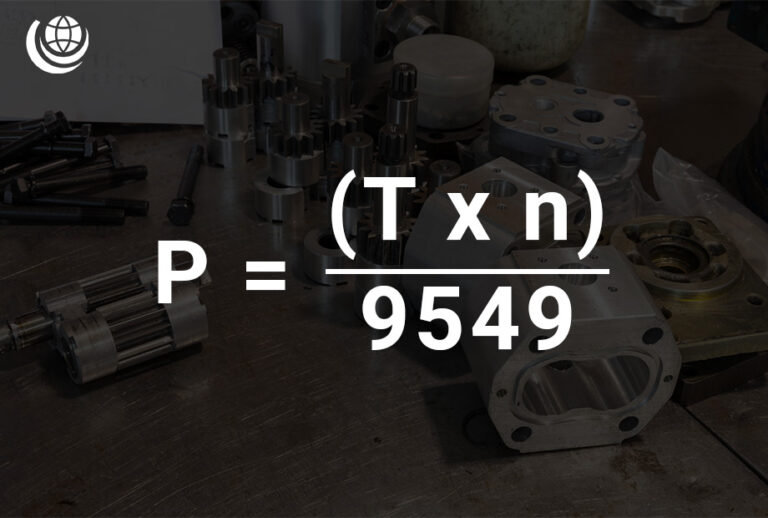 »
»
Power (kW)
P = (T x n) / 9549
When…
P = Power (kW)
T = Torque (Nm)
n = Speed (rpm)
9549 = Constant
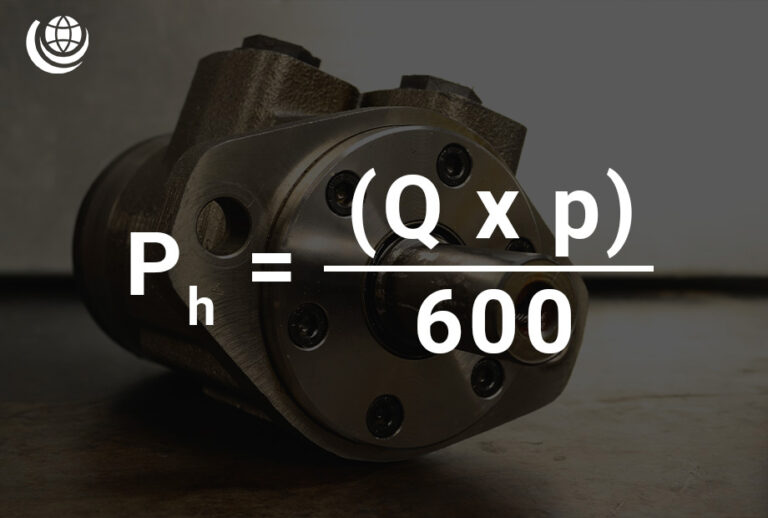 »
»
Power (Hydraulic)
Ph = (Q x p) / 600
when…
Ph = Hydraulic Power (kW)
Q = Flow Rate (l/min)
p = Pressure (bar)
600 = Conversion constant
 »
»
Torque
T = (D x p) / 20π
when…
T = Torque (Nm)
p = Pressure (bar)
D = Displacement (cm³/rev)
Constant = 20π
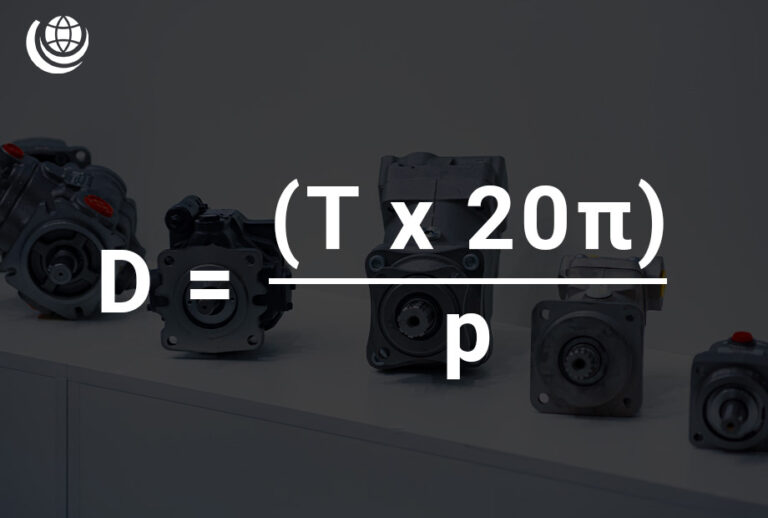 »
»
Displacement
D = (T x 20π) / p
when…
T = Torque (Nm)
p = Pressure (bar)
D = Displacement (cm³/rev)
Constant = 20π
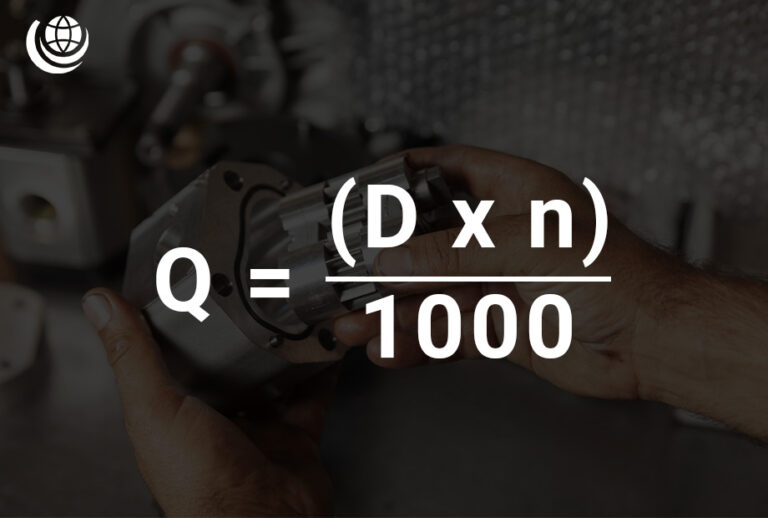 »
»
Flow Rate
Q = (D x n) / 1000
When…
Q = Flow Rate (l/min)
D = Pump Displacement (cm³/rev)
n = Speed (rpm)
1000 = Constant (converts cm³/min to litres/min)
 »
»
An introduction to the different types of hydraulic motors
Want to learn more about hydraulic motors? Our free introduction to the different kinds of motors is a great place to start!
Expand your hydraulic engineering knowledge further by visiting our Fluid Power Technical Knowledge Hub…
Hydraulic Formulas for Hydraulic Cylinders
When people imagine hydraulics systems, they probably picture articulated machines, powered by hydraulic cylinders.
Like muscles to hydraulic systems, cylinders are linear actuators that use hydraulic fluid to make light work of lifting, lowering or moving with massive amounts of force. Here are some formulas you can use to calculate important measurements relating to hydraulic cylinders.
 »
»
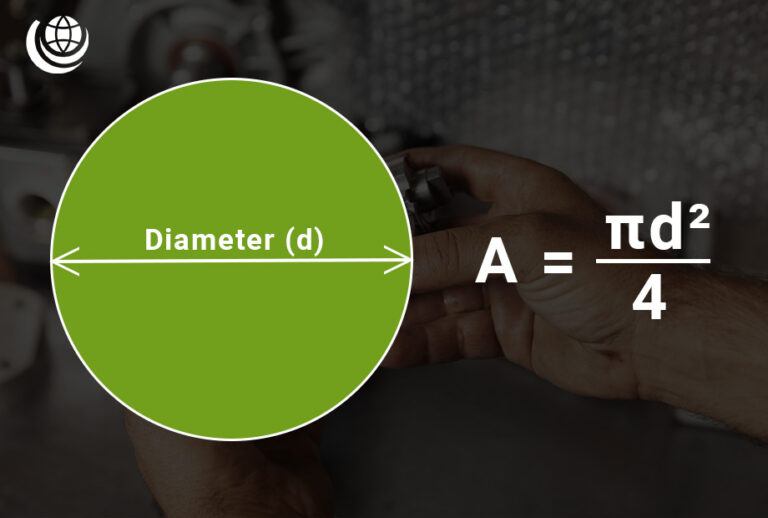
Piston Area
A = πd² / 4
Where…
A = Area (cm²)
d = Bore Diameter (cm)
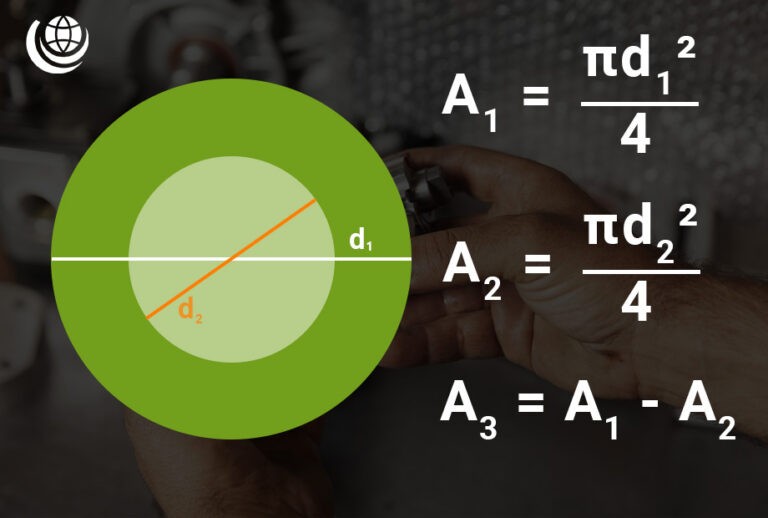 »
»
Annulus Area
A3 = A1 – A2
Where…
A1 = πd1² / 4 = Piston Area
A2 = πd2² / 4 = Rod Area
A3 = Annulus Area
 »
»
Force, Area and Pressure
F = p x A
p = F / A
A = F / p
When…
F = Force (N)
P = Pressure (Pa)
A = Area (m²)
 »
»
Speed, Flow and Area
Q = S x A
S = Q / A
A = Q / S
When…
S = Speed (cm/s)
Q = Flow Rate (cm³/min)
A = Area (cm²)
 »
»
The Ultimate Guide to Hydraulic Cylinders
When people imagine hydraulics systems, they probably picture articulated machines, powered by hydraulic cylinders.
Like muscles to hydraulic systems, cylinders are everywhere; but how do they work? And what are the different kinds?
 »
»
Hydraulic Cylinder Maintenance
Regular hydraulic cylinder maintenance is crucial to the upkeep and performance of your system.
Here we share our top tips for successful hydraulic cylinder maintenance.
Pressure Drop
When fluid moves through a hydraulic system, there is a gradual loss of pressure due to a number of factors such as pipeline resistance, and different components such as valves. This decrease in pressure is known as ‘pressure drop’ and can be calculated using the formulas below.
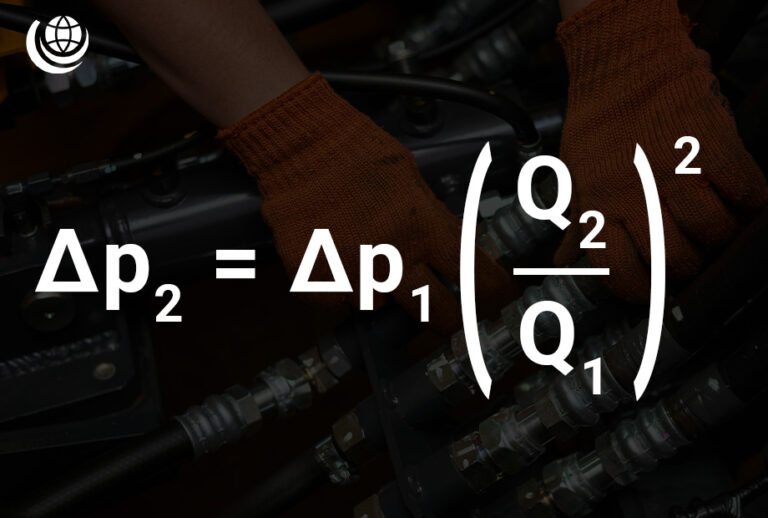 »
»
Pressure Drop at a Change of Flow
Δp2 = Δp1 (Q2 / Q1)²
When…
Δp1 = Pressure before Flow Change (bar)
Δp2 = Pressure after Change in Flow (bar)
Q1 = Original Flow (l/min)
Q2 = Flow after Change (l/min)
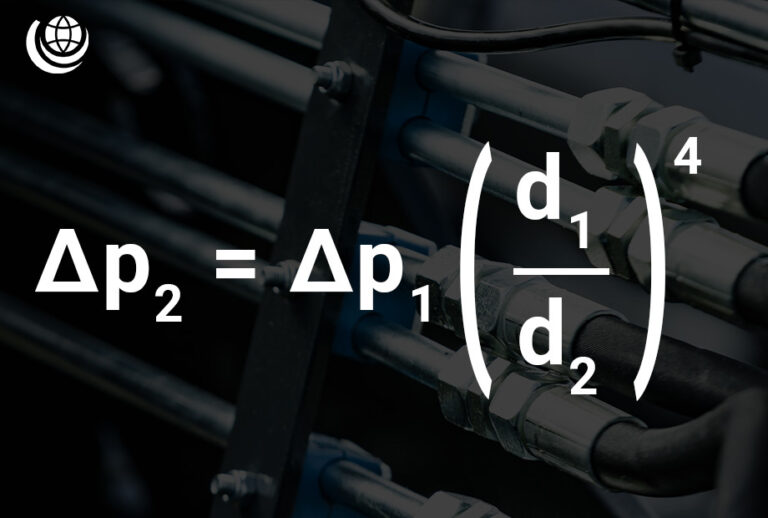 »
»
Pressure Drop at a Change of Diameter
Δp2 = Δp1 (d1 / d2)4
When…
Δp1 = Pressure before Flow Change (bar)
Δp2 = Pressure after Change in Flow (bar)
d1 = Original Diameter (mm)
d2 = Diameter after Change (mm)
 »
»
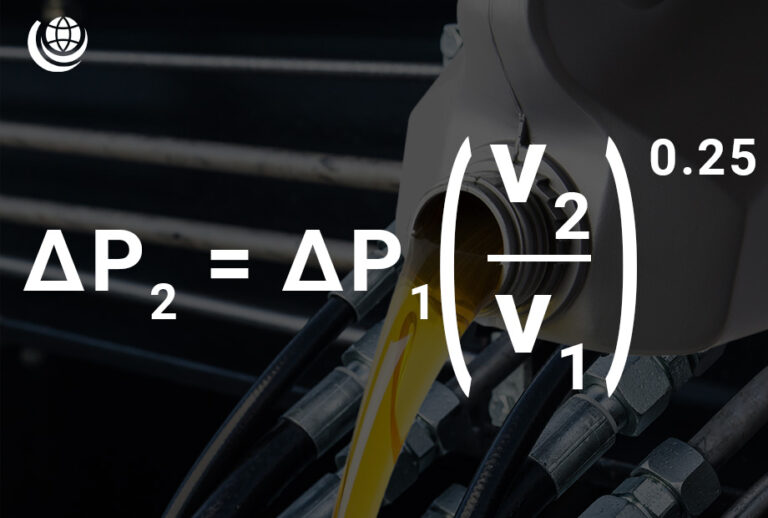
Pressure Drop at a Change of Viscosity
Δp2 = Δp1 (V2/ V1) 0.25
When…
Δp1 = Pressure before Flow Change (bar)
Δp2 = Pressure after Change in Flow (bar)
v1 = Original Viscosity (cSt)
v2 = Viscosity after Change (cSt)
Subscribe to our newsletter to receive more free technical guides, product insights and resources…




